Faulhaber's Formula

In mathematics, Faulhaber's formula, named after the early 17th century mathematician Johann Faulhaber, expresses the sum of the ''p''-th powers of the first ''n'' positive integers

:$\sum_^n k^p = 1^p + 2^p + 3^p + \cdots + n^p$

as a (''p'' + 1)th-degree polynomial function of ''n'', the coefficients involving Bernoulli numbers ''B_j'', in the form submitted by Jacob Bernoulli and published in 1713:

: $\sum_^n k^ = \frac+\fracn^p+\sum_^p \fracp^\underlinen^,$
where $p^\underline=(p)_=\dfrac$ is a falling factorial.

History

Faulhaber's formula is also called Bernoulli's formula. Faulhaber did not know the properties of the coefficients later discovered by Bernoulli. Rather, he knew at least the first 17 cases, as well as the existence of the Faulhaber polynomials for odd powers described below. The arxiv.org paper has a misprint in the formula for the sum of 11th powers, which was corrected in the printed version
Correct version.
/ref>

A rigorous proof of these formulas and Faulhaber's assertion that such formulas would exist for all odd powers took until , two centuries later.

Faulhaber polynomials

The term ''Faulhaber polynomials'' is used by some authors to refer to something other than the polynomial sequence given above. Faulhaber observed that if ''p'' is odd, then

:$1^p + 2^p + 3^p + \cdots + n^p$

is a polynomial function of

:$a=1+2+3+\cdots+n= \frac.$

In particular:

:$1^3 + 2^3 + 3^3 + \cdots + n^3 = a^2;$

:$1^5 + 2^5 + 3^5 + \cdots + n^5 = ;$

:$1^7 + 2^7 + 3^7 + \cdots + n^7 = ;$

:$1^9 + 2^9 + 3^9 + \cdots + n^9 = ;$

:$1^ + 2^ + 3^ + \cdots + n^ = .$

The first of these identities (the case ''p'' = 3) is known as Nicomachus's theorem.

More generally,

:
\begin
1^ + 2^ &+ 3^ + \cdots + n^\\ &= \frac \sum_^m \binom
(2-2^)~ B_ ~\left 8a+1)^-1\right
\end

Some authors call the polynomials in ''a'' on the right-hand sides of these identities Faulhaber polynomials. These polynomials are divisible by because the Bernoulli number is 0 for odd .

Faulhaber also knew that if a sum for an odd power is given by

:$\sum_^n k^ = c_1 a^2 + c_2 a^3 + \cdots + c_m a^$

then the sum for the even power just below is given by

:$\sum_^n k^ = \frac(2 c_1 a + 3 c_2 a^2+\cdots + (m+1) c_m a^m).$

Note that the polynomial in parentheses is the derivative of the polynomial above with respect to ''a''.

Since ''a'' = ''n''(''n'' + 1)/2, these formulae show that for an odd power (greater than 1), the sum is a polynomial in ''n'' having factors ''n''² and (''n'' + 1)², while for an even power the polynomial has factors ''n'', ''n'' + ½ and ''n'' + 1.

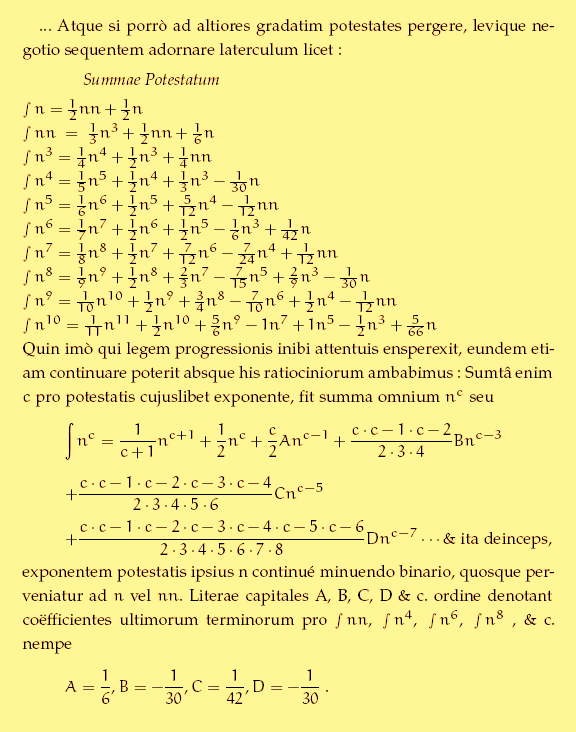
''Summae Potestatum''

In 1713, Jacob Bernoulli published under the title ''Summae Potestatum'' an expression of the sum of the powers of the first integers as a ()th-degree polynomial function of , with coefficients involving numbers , now called Bernoulli numbers:
: $\sum_^n k^ = \frac+\fracn^p+ \sum_^p B_j n^.$

Introducing also the first two Bernoulli numbers (which Bernoulli did not), the previous formula becomes
:$\sum_^n k^p = \sum_^p B_j n^,$
using the Bernoulli number of the second kind for which $B_1=\frac$, or
:$\sum_^n k^p = \sum_^p (-1)^j B_j n^,$
using the Bernoulli number of the first kind for which $B_1=-\frac.$

For example, as
:$B_0=1,~B_1=1/2,~B_2=1/6,~B_3=0,~B_4=-1/30,$
one has for ,
:$\begin1^4 + 2^4 + 3^4 + \cdots + n^4 &= \sum_^4 B_j n^\\ &= \left(B_0 n^5+5B_1n^4+10B_2n^3+10B_3n^2+5B_4n\right)\\ &= \fracn^5 + \fracn^4+ \fracn^3- \fracn.\end$

Faulhaber himself did not know the formula in this form, but only computed the first seventeen polynomials; the general form was established with the discovery of the Bernoulli numbers (see History section). The derivation of Faulhaber's formula is available in ''The Book of Numbers'' by John Horton Conway and Richard K. Guy.

There is also a similar (but somehow simpler) expression: using the idea of telescoping and the binomial theorem, one gets ''Pascal's identity'':
:$\begin(n+1)^-1 &= \sum_^n \left((m+1)^ - m^\right)\\ &= \sum_^k \binom (1^p+2^p+ \dots + n^p).\end$
This in particular yields the examples below – e.g., take to get the first example. In a similar fashion we also find
:$\beginn^ = \sum_^n \left(m^ - (m-1)^\right) = \sum_^k (-1)^\binom (1^p+2^p+ \dots + n^p).\end$

Examples

:$1 + 2 + 3 + \cdots + n = \frac = \frac$ (the triangular numbers)

:$1^2 + 2^2 + 3^2 + \cdots + n^2 = \frac = \frac$ (the square pyramidal numbers)

:1^3 + 2^3 + 3^3 + \cdots + n^3 = \left frac\right2 = \frac (the triangular numbers squared)

:$\begin 1^4 + 2^4 + 3^4 + \cdots + n^4 & = \frac \\ & = \frac \end$

:$\begin 1^5 + 2^5 + 3^5 + \cdots + n^5 & = \frac \\ & = \frac \end$

:$\begin 1^6 + 2^6 + 3^6 + \cdots + n^6 & = \frac \\ & = \frac \end$

From examples to matrix theorem

From the previous examples we get:
\begin
\sum_^n i^0 &= n \\ ex\sum_^n i^1 &= n+n^2 \\ ex\sum_^n i^2 &= n+n^2+n^3 \\ ex\sum_^n i^3 &= n^2+n^3+n^4 \\ ex\sum_^n i^4 &= -n+n^3+n^4+n^5 \\ ex\sum_^n i^5 &= -n^2+n^4+n^5+n^6 \\ ex\sum_^n i^6 &= n-n^3+n^5+n^6+n^7
\end
Writing these polynomials as a product between matrices gives
$\begin \sum_^ i^0 \\ \sum_^ i^1 \\ \sum_^ i^2 \\ \sum_^ i^3 \\ \sum_^ i^4 \\ \sum_^ i^5 \\ \sum_^ i^6 \\ \end = G_7 \begin n \\ n^2 \\ n^3 \\ n^4 \\ n^5 \\ n^6 \\ n^7 \\ \end \qquad \text\qquad G_7 = \begin 1& 0& 0& 0& 0&0& 0\\ & & 0& 0& 0& 0& 0\\ & && 0& 0& 0& 0\\ 0& & & & 0&0& 0\\ -& 0& & & &0& 0\\ 0& -& 0& & & & 0\\ & 0& -& 0& && \end$

Surprisingly, inverting the matrix of polynomial coefficients yields something more familiar:
$G_7^=\begin 1& 0& 0& 0& 0& 0& 0\\ -1& 2& 0& 0& 0& 0& 0\\ 1& -3& 3& 0& 0& 0& 0\\ -1& 4& -6& 4& 0& 0& 0\\ 1& -5& 10& -10& 5& 0& 0\\ -1& 6& -15& 20& -15& 6& 0\\ 1& -7& 21& -35& 35& -21& 7\\ \end = \overline_7$

In the inverted matrix, Pascal's triangle can be recognized, without the last element of each row, and with alternating signs.

Let $A_7$ be the matrix obtained from $\overline_7$ by changing the signs of the entries in odd diagonals, that is by replacing $a_$ by $(-1)^ a_$, let $\overline_7$ be the matrix obtained from $G_7$ with a similar transformation, then
$A_7=\begin 1& 0& 0& 0& 0& 0& 0\\ 1& 2& 0& 0& 0& 0& 0\\ 1& 3& 3& 0& 0& 0& 0\\ 1& 4& 6& 4& 0& 0& 0\\ 1& 5& 10& 10& 5& 0& 0\\ 1& 6& 15& 20& 15& 6& 0\\ 1& 7& 21& 35& 35& 21& 7\\ \end \quad A_7^=\begin 1& 0& 0& 0& 0&0& 0\\ -& & 0& 0& 0& 0& 0\\ & -&& 0& 0& 0& 0\\ 0& & -& & 0&0& 0\\ -& 0& & -& &0& 0\\ 0& -& 0& & -& & 0\\ & 0& -& 0& &-& \end=\overline_7$
and also
$\begin \sum_^ i^0 \\ \sum_^ i^1 \\ \sum_^ i^2 \\ \sum_^ i^3 \\ \sum_^ i^4 \\ \sum_^ i^5 \\ \sum_^ i^6 \\ \end = \overline_7\begin n \\ n^2 \\ n^3 \\ n^4 \\ n^5 \\ n^6 \\ n^7 \\ \end$
This is because it is evident that
$\sum_^i^m-\sum_^i^m=n^m$ and that therefore polynomials of degree $m+ 1$ of the form $\fracn^+\fracn^m+\cdots$ subtracted the monomial difference $n^m$ they become $\fracn^-\fracn^m+\cdots$.

This is true for every order, that is, for each positive integer , one has $G_m^ = \overline_m$ and $\overline_m^ = A_m.$
Thus, it is possible to obtain the coefficients of the polynomials of the sums of powers of successive integers without resorting to the numbers of Bernoulli but by inverting the matrix easily obtained from the triangle of Pascal.

Proof with exponential generating function

Let
:$S_(n)=\sum_^ k^p,$
denote the sum under consideration for integer $p\ge 0.$

Define the following exponential generating function with (initially) indeterminate $z$
:$G(z,n)=\sum_^ S_(n) \fracz^p.$
We find
:$\begin G(z,n) =& \sum_^ \sum_^ \frac(kz)^p =\sum_^e^=e^\cdot\frac,\\ =& \frac. \end$
This is an entire function in $z$ so that $z$ can be taken to be any complex number.

We next recall the exponential generating function for the Bernoulli polynomials $B_j(x)$
:$\frac=\sum_^ B_j(x) \frac,$
where $B_j=B_j(0)$ denotes the Bernoulli number with the convention $B_=-\frac$. This may be converted to a generating function with the convention $B^+_1=\frac$ by the addition of $j$ to the coefficient of $x^$ in each $B_j(x)$ ($B_0$ does not need to be changed):
:$\begin \sum_^ B^+_j(x) \frac =& \frac+\sum_^jx^\frac\\ =& \frac+\sum_^x^\frac\\ =& \frac+ze^\\ =& \frac\\ =& \frac \end$
It follows immediately that
:$S_p(n)=\frac$
for all $p$.

Alternate expressions

* By relabelling we find the alternative expression $\sum_^n k^p= \sum_^p B_ n^.$
* We may also expand $G(z,n)$ in terms of the Bernoulli polynomials to find $\begin G(z,n) &= \frac - \frac\\ &= \sum_^ \left(B_j(n+1)-(-1)^j B_j\right) \frac, \end$ which implies $\sum_^n k^p = \frac \left(B_(n+1)-(-1)^B_\right) = \frac\left(B_(n+1)-B_(1) \right).$ Since $B_n = 0$ whenever $n > 1$ is odd, the factor $(-1)^$ may be removed when $p > 0$.
* It can also be expressed in terms of Stirling numbers of the second kind and falling factorials asConcrete Mathematics, 1st ed. (1989), p. 275. $\sum_^n k^p = \sum_^p \left\\frac,$ $\sum_^n k^p = \sum_^ \left\\frac.$ This is due to the definition of the Stirling numbers of the second kind as mononomials in terms of falling factorials, and the behaviour of falling factorials under the indefinite sum.
* Faulhaber's formula was generalized by Guo and Zeng to a -analog.

Relationship to Riemann zeta function

Using $B_k=-k\zeta(1-k)$, one can write
:$\sum\limits_^n k^p = \frac - \sum\limits_^\zeta(-j)n^.$

If we consider the generating function $G(z,n)$ in the large $n$ limit for $\Re (z)<0$, then we find
:$\lim_G(z,n) = \frac=\sum_^ (-1)^B_j \frac$
Heuristically, this suggests that
:$\sum_^ k^p=\frac.$
This result agrees with the value of the Riemann zeta function $\zeta(s)=\sum_^\frac$ for negative integers $s=-p<0$ on appropriately analytically continuing $\zeta(s)$.

Umbral form

In the classical umbral calculus one formally treats the indices ''j'' in a sequence ''B''_''j'' as if they were exponents, so that, always considering the variant $B_1= \frac$, in this case we can apply the binomial theorem and say
\begin
\sum_^n k^p &= \sum_^p B_j n^ \\ ex& = \sum_^p B^j n^ \\ ex&= .
\end

In the ''modern'' umbral calculus, one considers the linear functional ''T'' on the vector space of polynomials in a variable ''b'' given by
$T(b^j) = B_j.$

Then one can say
\begin
\sum_^n k^p &= \sum_^p B_j n^ \\ ex&= \sum_^p T(b^j) n^ \\ ex&= T\left(\sum_^p b^j n^ \right) \\ ex&= T\left(\right).
\end

See also

*Polynomials calculating sums of powers of arithmetic progressions

Notes

External links

*
*
* A very rare book, but Knuth has placed a photocopy in the Stanford library, call number QA154.8 F3 1631a f MATH. ()
* (Winner of
Lester R. Ford Award

*

*{{Cite news
, last=Orosi
, first=Greg
, year=2018
, title=A Simple Derivation Of Faulhaber's Formula
, url=http://www.math.nthu.edu.tw/~amen/2018/AMEN-170803.pdf
, periodical = Applied Mathematics E-Notes
, volume =18
, pages =124–126

A visual proof for the sum of squares and cubes

Finite differences